Basic HTML Version
391
IBRACON Structures and Materials Journal • 2012 • vol. 5 • nº 3
P. T. C. MENDES | M. L. T. MOREIRA
|
P. M. PIMENTA
for each of the finite elements constituent to the model. For un-
cracked section was considered as a whole with perfect adher-
ence between representative elements of concrete and the steel
bars. For cracked section, the height of the neutral axis was
estimated and the crack of the section was simulated with the
removal of the concrete elements below the neutral axis in the
vicinity of the cracks and in the other sections remained in the
same conditions as in uncracked section.
2.2 Modulus of elasticity of concrete and steel
For the analysis of the models a module of elasticity on the con-
crete E
c
= 23.8 GPa was adopted corresponding to the suggested
value in the NBR 6118 [ 3 ].
Due to the scarcity of information relative to the mechanical char-
acteristics of the concrete used on these bridges, it was chosen
to analyze them considering the module of elasticity of the cor-
responding concrete at 50% of the E
c
value with the objective of
evaluating the influence of this factor in the distribution of the con-
crete and steel stresses. The module of elasticity of the steel was
considered E
s
= 210.0 GPa.
Figure 6. For analysis effect concrete with f
ck
= 18.0 MPa was ad-
mitted and reinforcement constituted of steel CA24 or CA50.
2.1 Computational models
The SAP2000-V11 program was used for the numerical evalu-
ation of the bridge behavior. The first model consisted in the
discretization of beams with finite elements of bar and the slabs
with finite elements of shell (B-S), according to Figure 7. In this
model, under bending moments acting on the longitudinal beams,
the stresses on the concrete and in the reinforcement were ob-
tained from the admission of Navier’s hypothesis in maintenance
of the plane section, in uncracked and cracked cross section,
considering the resulting tensile in the reinforcement situated in
its center of gravity. In the second model, the constituents of the
superstructure of the bridge were discretizated with solid finite
elements (SOL) representative of concrete and the different re-
inforced bars, with their mechanical characteristics, according to
Figure 8. In this case the stresses in the concrete and in several
bars of the reinforcement were provided directly by the program
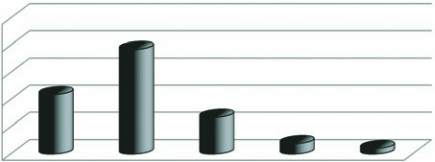
Figure 5 – Distribution of bridges TB240 by
groups of maximum span, excluding
the ones not informed
0
50
100
150
200
250
SPAN < 10m
10m
≤
SPAN < 20m
20m
≤
SPAN < 30m
30m
≤
SPAN < 40m
OTHER
109;27,5%
192;48,4%
67;16,9%
19;4,8%
10;2,5%
DISTRIBUTION OF BRIDGES TB240 BY GROUPS OF
MAXIMUN SPAN
Figure 6 – Representative bridge (MENDES [1] )
Figure 7 – Model with finite elements of bars
and shells (MENDES [ 1 ])
Figure 8 – Model with solid finite elements
(MENDES [1] )

