Basic HTML Version
144
IBRACON Structures and Materials Journal • 2013 • vol. 6 • nº 1
The strut-and-tie models in reinforced concrete structures analysed by a numerical technique
ments were used (mesh 150x47). Figure [7a] show the optimal
design obtained by the SESO formulation and compared with the
work developed by Liang [7], Figure [7b], and Schlaich
et al
. [1],
Figure [7c].
The optimum topology design shown in Figure [7a] was obtained
with a final volume of 30.3%; dark and light regions respectively
indicate the compressed regions, strut, and tensioned regions, tie.
Figure [7b] shows the optimal design presented by [7], who uses
the ESO optimization method to obtain a final volume of 33%. Fig-
ure [7c] shows the optimal configuration for the strut-and-tie design
proposed by [1], who uses the strut-and-tie model. The graph in
Figure [8] shows the monitoring made by this formulation to deter-
mine the optimal topology. The growth of the PI values is plotted
for each iteration path, and the sharp drop in PI indicates that the
previous iteration is thus the area of optimal design.
Schlaich
et al
. [1] proposed strengthening for this structure, ob-
tained with the use of a strut-and-tie model deriving from the com-
bination of the finite element method with a procedure to obtain the
flow strength, using the method called “load path”. Thus, Figure
[12] shows the disposition of reinforcement by the authors, [1], to
strengthen the beam cavity.
With the indication of optimal topology obtained by the present for-
mulation, a proposal for a strut-and-tie model can be directly pre-
sented. Note the proposed strut-and-tie shown in Figure [9] where
the dotted and continuous lines indicate, respectively, compressed
members (C), strut, and tensioned members (T), tie. The efforts at
the members where the flow stress stand out can be calculated by
multiplying the average stress values of each member and their re-
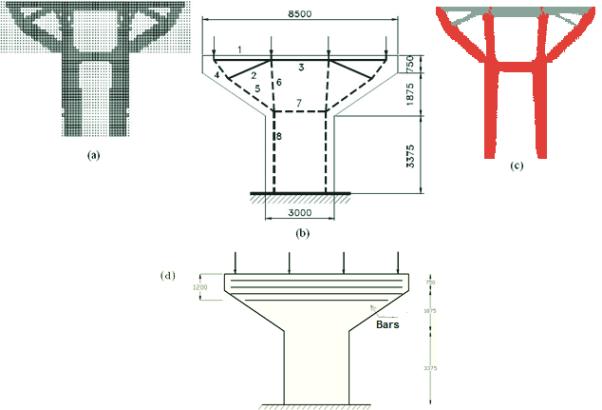
Figure 5 – (a) Optimal topology (b) strut-and-tie model, proposed by [6], mm; (c) Optimal topology
using the present model; (d) Proposed disposition of reinforcement for the present model (mm)
Table 1 – Strut-and-tie forces (kN) for each
member of the bridge
Member
Force [6]
Force
(present model)
1
2,114
2,192
2
1,162
1,195
3
3,363
3,454
4
-3,470
-3,589
5
-3,919
-4,083
6
-3,219
-3,482
7
-3,363
-3,569
8
-5,500
-5,964
Figure 6 – Domain design (mm), [7]

